二分图（例题）

· 浏览次数 : 0

小编点评

The code is an implementation of the Hungarian algorithm to determine the maximum number of connected components in a given graph. **Key Concepts:** * **Hungarian Algorithm:** An algorithm to find the maximum number of connected components in a graph. * **Graph:** A collection of connected vertices. * **Connected Component:** A group of vertices where any two vertices are connected by an edge. * **Component:** A group of connected vertices that form a subset of the graph. **Algorithm Steps:** 1. **Input:** - Read the input data, including the number of vertices in the graph, the graph itself (represented as a string), and the maximum edge weight. 2. **Initialization:** - Initialize a 2D vector `match` to keep track of which vertices belong to which component. - Initialize an array of vectors `s` to store the components of the graph. 3. **Depth-First Search (DFS):** - For each vertex in the graph, perform a DFS to find all its connected vertices. - For each connected vertex, update the `match` array to indicate that it belongs to the same component as the current vertex. 4. **Counting Connected Components:** - After completing the DFS, count the number of components by counting the number of elements in the `match` array that are set to 1. 5. **Output:** - Print the number of connected components found in the graph. **Additional Notes:** * The code uses a 2D array `s` to represent the graph, where `s[i][j]` represents whether vertex `i` is connected to vertex `j`. * The code assumes that the graph is connected and has no cycles. * The time complexity of the algorithm is O(V + E), where V is the number of vertices and E is the number of edges in the graph.

正文

    https://www.cnblogs.com/kuangbiaopilihu/p/18184536

$\quad $ 这里不再介绍二分图的基础知识，只是一些例题的解释。

$\quad $ 当然，这道题可以用二分+并查集来解决。但这是二分图专辑，所以介绍一下二分图做法。
$\quad $ 首先如果两个罪犯之间有仇恨，那么当他们不在同一所监狱时不会发生冲突。若要若干个罪犯之间不产生冲突，那么将有仇恨的罪犯连边，则不会发生冲突的罪犯恰好形成一个二分图。
$\quad $ 所以按照有仇恨罪犯之间的怒气值排序，再二分一下答案下标，把边权大于二分答案的边加进去，如果形成了一个二分图，则答案合法。然后便可得出答案。

点击查看代码

  #include<bits/stdc++.h>
  using namespace std;
  const int N=1e5+100;
  vector<int> sl[N];
  struct stu{
      int x,y,w;
  }s[N];
  int col[N],n,m,ans;
  bool is_gragh(int cur,int fa,int color){
      col[cur]=color;
      for(int i=0;i<sl[cur].size();i++){
          int y=sl[cur][i];
          if(col[y]==color)return false;
          if(col[y]==0&&!is_gragh(y,cur,3-color))return false;
      }
      return true;
  }
  bool check(int x){
      for(int i=1;i<=n;i++)sl[i].clear();
      memset(col,0,sizeof col);
      for(int i=x+1;i<=m;i++){
          int x=s[i].x,y=s[i].y;
          sl[x].push_back(y);
          sl[y].push_back(x);
      }
      for(int i=1;i<=n;i++)if(col[i]==0)if(!is_gragh(i,0,1))return false;
      return true;
  }
  bool cmp(stu a,stu b){return a.w<b.w;}
  int main(){
      scanf("%d%d",&n,&m);
      for(int i=1;i<=m;i++)scanf("%d%d%d",&s[i].x,&s[i].y,&s[i].w);
      sort(s+1,s+1+m,cmp);
      int l=0,r=m;
      while(l<=r){
          int mid=(l+r)>>1;
          if(check(mid))r=mid-1,ans=mid;
          else l=mid+1;
      }
      printf("%d",s[ans].w);
      return 0;
  }

$\quad $ 可以发现，如果选择了一列，那么处于这一列的点将都被消除，那么就可以将该点与其所在行与所在列相连，以表示其关联。先拿样例举例：

$\quad $ 我们发现，点只存在于行和列之间的边上，那么将点省去，可以得到一个二分图。这样问题就变为了一个二分图的点最大覆盖问题，求最大匹配即可。

点击查看代码



  #include<bits/stdc++.h>
  using namespace std;
  const int N=1e4+100;
  bool vis[N];
  int n,k,match[N];
  vector<int> s[N<<1];
  bool dfs(int x){
      for(int i=0;i<s[x].size();i++){
          int y=s[x][i];
          if(!vis[y]){
              vis[y]=1;
              if(!match[y]||dfs(match[y])){
                  match[y]=x;
                  return true;
              }
          }
      }
      return false;
  }
  int Hungary(){
      int ans=0;
      for(int i=1;i<=n;i++){
          memset(vis,0,sizeof vis);
          if(dfs(i))ans++;
      }
      return ans;
  }
  int main(){
      scanf("%d%d",&n,&k);
      n<<=1;
      for(int i=1;i<=k;i++){
          int x,y;
          scanf("%d%d",&x,&y);
          y+=n;
          s[x].push_back(y);
          s[y].push_back(x);
      }
      printf("%d",Hungary());
      return 0;
  }

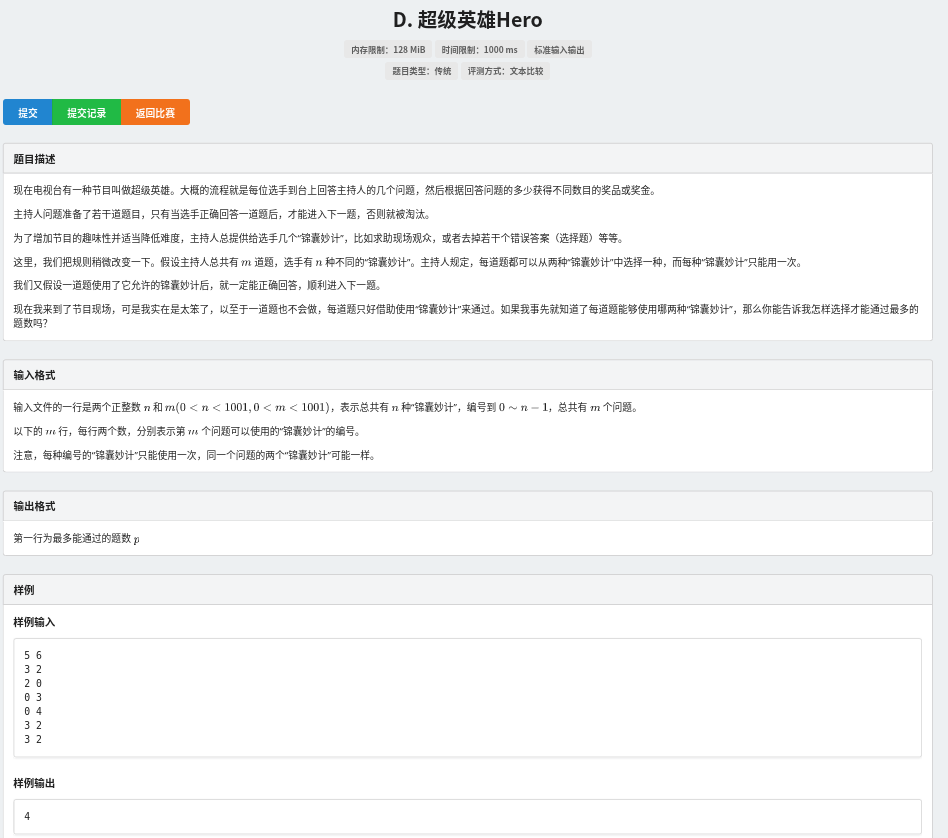
$\quad $ 还是先膜样例，这里用汉字表示锦囊，阿拉伯数字表示题目。

$\quad $ 同样可以得到一张二分图，只不过这道题不是要求最大匹配，因为答题出现错误就淘汰了，仔细观察匈牙利算法代码，可以发现他正是从1顺序开始寻找的，所以我们只要在无法匹配时打断循环即可。

点击查看代码



  #include<bits/stdc++.h>
  using namespace std;
  const int N=1e4+100;
  bool vis[N];
  int n,k,match[N];
  vector<int> s[N<<1];
  bool dfs(int x){
      for(int i=0;i<s[x].size();i++){
          int y=s[x][i];
          if(!vis[y]){
              vis[y]=1;
              if(!match[y]||dfs(match[y])){
                  match[y]=x;
                  return true;
              }
          }
      }
      return false;
  }
  int Hungary(){
      int ans=0;
      for(int i=1;i<=n;i++){
          memset(vis,0,sizeof vis);
          if(dfs(i))ans++;
          else break;
      }
      return ans;
  }
  int main(){
      scanf("%d%d",&n,&k);
      for(int i=1;i<=k;i++){
          int x,y;
          scanf("%d%d",&x,&y);
          x++,y++;
          s[i].push_back(y);
          s[i].push_back(x);
      }
      printf("%d",Hungary());
      return 0;
  }

$\quad $ 先求出所有小衫到所有出口所需时间，对时间小于k的情况，就将两者相连，最后还是的到一张二分图，此时只需要求出最大匹配即可。
注意开double！！

点击查看代码

  #include<bits/stdc++.h>
  using namespace std;
  const int N=1e4+100;
  bool vis[N];
  int n,k,match[N],m;
  vector<int> s[N<<1];
  double x[N],y[N];
  bool dfs(int x){
      for(int i=0;i<s[x].size();i++){
          int y=s[x][i];
          if(!vis[y]){
              vis[y]=1;
              if(!match[y]||dfs(match[y])){
                  match[y]=x;
                  return true;
              }
          }
      }
      return false;
  }
  int Hungary(){
      int ans=0;
      for(int i=1;i<=m;i++){
          memset(vis,0,sizeof vis);
          if(dfs(i))ans++;
      }
      return ans;
  }
  int main(){
      scanf("%d%d%d",&m,&n,&k);
      //m是小衫个数，n是点数，k是边权最大值。
      for(int i=1;i<=n;i++)scanf("%lf%lf",&x[i],&y[i]);
      for(int i=1;i<=m;i++){
          double xl,yl,vl,tl;
          scanf("%lf%lf%lf",&xl,&yl,&vl);
          for(int j=1;j<=n;j++){
              tl=sqrt((x[j]-xl)*(x[j]-xl)+(y[j]-yl)*(y[j]-yl));
              tl/=vl;
              // cout<<tl<<endl;
              if(k>=tl)s[i].push_back(j+m),s[j+m].push_back(i);
          }
      }
      printf("%d",Hungary());
      return 0;
  }

$\quad $ 这道题和穿越小行星群很像，但是有石头阻拦，对于有石头阻拦的，我们可以将一行视为两行、一列视为两列，再将合法的位置与其行列连边。这样又得到一张二分图，再求最大匹配即可。

点击查看代码

#inclu  de<bits/stdc++.h>
  using namespace std;
  const int N=65;
  char ch[N*N][N*N];
  bool vis[N*N];
  int n,m,match[N*N],row[N*N][N*N],col[N*N][N*N];
  int ntot,ltot;
  vector<int>s[N*N];
  bool dfs(int x){
      for(int i=0;i<s[x].size();i++){
          int y=s[x][i];
          if(!vis[y]){
              vis[y]=1;
              if(!match[y]||dfs(match[y])){
                  match[y]=x;
                  return true;
              }
          }
      }
      return false;
  }
  int Hungary(){
      int ans=0;
      for(int i=1;i<=ntot;i++){
          memset(vis,0,sizeof vis);
          if(dfs(i))ans++;
      }
      return ans;
  }
  int main(){
      scanf("%d%d",&m,&n);
      for(int i=1;i<=m;i++)scanf("%s",ch[i]+1);
      for(int i=1;i<=m;i++){
          for(int j=1;j<=n;j++){
              if(ch[i][j]-'#'){
                  if(j>1&&ch[i][j-1]-'#')row[i][j]=row[i][j-1];
                  else row[i][j]=++ntot;
                  if(i>1&&ch[i-1][j]-'#')col[i][j]=col[i-1][j];
                  else col[i][j]=++ltot;
              }

          }
      }
      for(int i=1;i<=m;i++){
          for(int j=1;j<=n;j++){
              if(ch[i][j]=='o')s[row[i][j]].push_back(col[i][j]+ntot);
          }
      }
      printf("%d",Hungary());
  }