在>1的整数中,如果只包含1和本身这两个约数,就被称为质数(素数)
\(O(n)\)
bool isprime(int x) {
if (x < 2) return false;
for (int i = 2; i < x; i++)
if (x % i == 0) return false;
return true;
}
复制约数 d 与 n / d 成对出现,可以枚举较小的那一个 \(O(\sqrt{n})\)
\(d <= n/d \\ d^2 <= n \\ d <= \sqrt{n}\)
难点
#include <iostream>
using namespace std;
bool isprime(int n) {
if (n < 2) return false;
for (int i = 2; i <= n / i; i++)
if (n % i == 0) return false;
return true;
}
int main() {
int n;
cin >> n;
while (n--) {
int x;
cin >> x;
if (isprime(x))
cout << "Yes" << endl;
else
cout << "No" << endl;
}
}
复制质因数指能整除给定正整数的质数。把一个合数分解成若干个质因数的乘积的形式,即求质因数的过程叫做分解质因数。
相关理论证明可看 数论——质数:分解质因数 - 知乎 (zhihu.com)
从2到\(\sqrt{n}\)枚举n的所有质因数,求其指数并输出。还要考虑最多有一个质因素大于\(\sqrt{n}\)的情况,单独判断输出。 最坏 \(O(\sqrt{n})\),最好 \(O(logn)\) (考虑\(2^k\)情况)
#include <iostream>
using namespace std;
void divide(int n) {
for (int i = 2; i <= n / i; i++) {
if (n % i == 0) {
int cnt = 0;
while (n % i == 0) {
cnt++;
n /= i;
}
cout << i << " " << cnt << endl;
}
}
if (n > 1) cout << n << " " << 1 << endl;
}
int main() {
int n;
cin >> n;
while (n--) {
int x;
cin >> x;
divide(x);
cout << endl;
}
}
复制朴素算法是从前往后删倍数(2~p-1都不是n的约数,所以n是质数)
调和级数\(1/2+1/3+1/4+1/5+...+1/∞\) 极限等于 \(lnn+C\)。
\(lnn < log_2n\),因此朴素算法复杂度为 \(O(nlogn)\)
埃式筛法:只删除2~p-1中质数的倍数,原理跟867类似(算数基本定理:每个正整数都可以唯一表示成素数的乘积)
粗略估计:1~n当中,有\(n/lnn\)个质数,时间复杂度变为 \(O(n)\),真实复杂度 \(O(nloglogn)\),两者差不多一个级别
#include <algorithm>
#include <iostream>
using namespace std;
const int N = 1e6 + 10;
int primes[N], cnt;
bool st[N];
void get_primes(int n) {
for (int i = 2; i <= n; i++) {
if (!st[i]) {
primes[cnt++] = n;
for (int j = i + i; j <= n; j += i) st[j] = true;
}
}
}
int main() {
int n;
cin >> n;
get_primes(n);
cout << cnt << endl;
return 0;
}
复制线性筛法,\(O(n)\),基本思路一样(基于每个质数的倍数为非质数),当 n 很大时,速度比埃式筛法快一倍。
每个数只会被其最小质因子筛掉
#include <algorithm>
#include <iostream>
using namespace std;
const int N = 1e6 + 10;
int primes[N], cnt;
bool st[N];
void get_primes(int n) {
for (int i = 2; i <= n; i++) {
if (!st[i]) primes[cnt++] = i;
for (int j = 0; primes[j] * i <= n; j++) {
st[primes[j] * i] = true;
if (i % primes[j] == 0) break; // primes[j] 一定是 i 的最小质因子
}
}
}
int main() {
int n;
cin >> n;
get_primes(n);
cout << cnt << endl;
return 0;
}
复制与866优化原理类似 \(O(\sqrt{n})\)
#include <algorithm>
#include <iostream>
#include <vector>
using namespace std;
vector<int> get_divisors(int n) {
vector<int> res;
for (int i = 1; i <= n / i; i++) {
if (n % i == 0) {
res.push_back(i);
if (i != n / i) res.push_back(n / i); // 避免平方
}
}
sort(res.begin(), res.end());
return res;
}
int main() {
int n;
cin >> n;
while (n--) {
int x;
cin >> x;
auto res = get_divisors(x);
for (auto t : res) cout << t << ' ';
cout << endl;
}
}
复制
利用算术基本定理,每个质因数有(1+n)种选择。m个选择组合得出m个约数
具体原理可看 第四章 数学知识(一)——质数与约数 - 知乎 (zhihu.com)
INT_MAX 约数个数约1500
求 n 个数的乘积的约数个数,可以求每个数的每个质因子指数之和,然后套用公式。
#include <algorithm>
#include <iostream>
#include <unordered_map>
using namespace std;
typedef long long LL;
const int mod = 1e9 + 7;
int main() {
int n;
cin >> n;
unordered_map<int, int> primes;
while (n--) {
int x;
cin >> x;
for (int i = 2; i <= x / i; i++) {
while (x % i == 0) {
x /= i;
primes[i]++;
}
}
if (x > 1) primes[x]++;
}
LL res = 1;
for (auto prime : primes) res = res * (prime.second + 1) % mod;
cout << res << endl;
return 0;
}
复制#include <algorithm>
#include <iostream>
#include <unordered_map>
using namespace std;
typedef long long LL;
const int mod = 1e9 + 7;
int main() {
int n;
cin >> n;
unordered_map<int, int> primes;
while (n--) {
int x;
cin >> x;
for (int i = 2; i <= x / i; i++) {
while (x % i == 0) {
x /= i;
primes[i]++;
}
}
if (x > 1) primes[x]++;
}
LL res = 1;
for (auto prime : primes) {
int p = prime.first, a = prime.second;
LL t = 1;
while (a--) {
t = (t * p + 1) % mod;
}
res = res * t % mod;
}
cout << res << endl;
return 0;
}
复制欧几里得算法(辗转相除法)
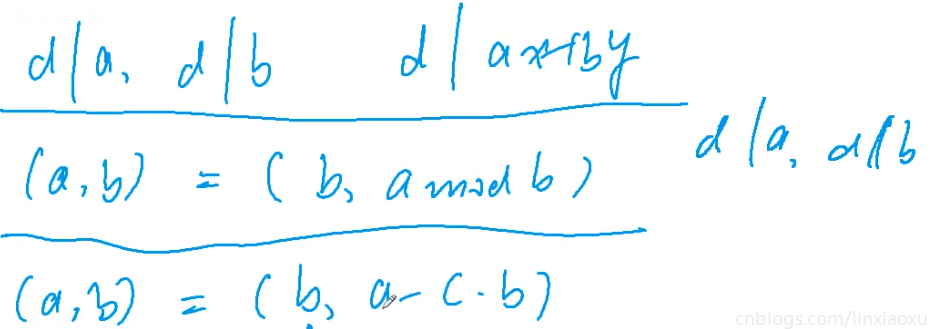
#include <algorithm>
#include <iostream>
#include <unordered_map>
using namespace std;
// a 和 0 的最大公约数是 a
int gcd(int a, int b) { return b ? gcd(b, a % b) : a; }
int main() {
int n;
cin >> n;
while (n--) {
int a, b;
cin >> a >> b;
cout << gcd(a, b) << endl;
}
return 0;
}
复制